The puzzle grid
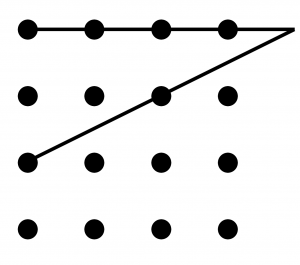
Here is a puzzle I enjoy giving to students. Given a 4×4 grid of sixteen dots, draw six straight lines that form a continuous path passing through all of the dots. Here, continuous means you must be able to draw over your six lines in one go without taking your pen off the paper.
This task is easy to complete with seven lines and impossible with five. Six is where the interesting puzzle lies.
I have given this to undergraduate students up and down the UK, and I have noticed a common response that makes me enjoy this puzzle and the lesson it teaches.
Many students start playing with the puzzle, drawing lines on grids right away. This is good because playing is how you start to understand puzzles and by showing their thought processes I can look out for when they have misunderstood the problem. In my experience, this is a problem often misunderstood.
One approach I see quite often is to draw lines within the grid always moving to the next adjacent dot – either up, down, left, right or diagonally. I ask the student, why aren’t you drawing outside the grid? “What,” the student typically replies, “you’re allowed to go outside the grid?”
Going outside of the grid on a strange diagonal
Then I ask, why are you only moving to adjacent dots, why not move at strange diagonals and jump around the grid in a more irregular fashion? Again, the response: “is that allowed?”
The statement of the problem was: “draw six straight lines that form a continuous path passing through all of the dots”. This says nothing to limit how you can move through the dots or where on the page you can draw your lines.
In fact, I know of fourteen solutions to this problem and each of them involves a strange diagonal and leaving the confines of the grid. By imagining these additional conditions the student creates a new problem – one that cannot be solved.
Good puzzles lie on a thin line between easy and impossible, and the particular wording in the statement of the problem is likely to be very carefully chosen to give you enough to solve it without being too easy. A would-be puzzle solver must check the wording to be sure they are attempting the puzzle that has actually been set and not some imagined, impossible version.